4200.25 – Sighting an Airplane

You are standing outside on a beautiful clear day. An airplane at 3000 feet files over head at 200 mph. You don't notice this until you hear the plane, but the instant that happens you spot the plane at $x$ degrees above the horizon. The sound you are hearing, however, came from the plane somewhat earlier. At that time it was 20 degrees above the horizon.

Find $x$ , assuming that $\dfrac{1100 \ \ft}{\sec}$ is the speed of sound.

Solution

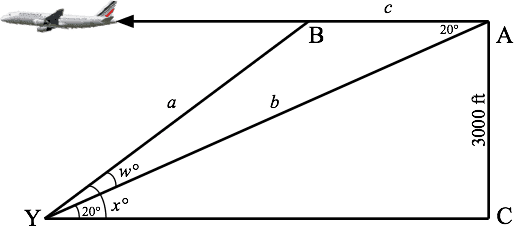
Referring to the diagram: You are at point $Y$ . When you first heard the plane it was at $B$ but the sound arrived from $A$ . The plane flew from $A$ to $B$ while the sound traveled to you, that is, traveled from $A$ to $Y$ . Our strategy is to find $a$ , $b$ and $c$ , concentrating on $\triangle ABY$ . Once we find $w$ we can find $x = w + 20$ .

So,

$\begin{align*}\dfrac{3000 \ \ft}{b} &= \sin(20^\circ) \\b &= \dfrac{3000 \ \ft}{\sin(20^\circ)} \\[1em]&\approx 8771 \ \ft\end{align*}$

At $\dfrac{1100 \ \ft}{\sec}$ the sound took 7.97 seconds to get to $Y$ . In that time, the plane flew from $A$ to $B$ at $\dfrac{200 \text{ mi}}{\text{hr}}$ . So

$\begin{align*}c &= AB \\&= \left(\dfrac{200}{3600}\right) \times 7.97 \\&= .443 \text{ mi.} \\&\approx 2339 \ \ft\end{align*}$

So much for $b$ and $c$ . We now get $w$ by first finding $a$ . Using the law of cosines:

$\begin{align*}a^2 &= 2339^2 + 8771^2 - 2 (2339) (8771) \cos(20^\circ) \\&= 43845080 \\a &\approx 6622 \ \ft\end{align*}$

Then, using the law of sines:

$\begin{align*}\sin w &= \dfrac{c \sin(20^\circ)}{a} \\[1em]&\approx \dfrac{2339 \sin(20^\circ)}{6622} \\[1em]&= 0.1208 \\[0.5em]w &\approx 6.94^\circ\end{align*}$

Thus $x \approx 26.94^\circ$ .

Note: This problem is presented implausibly as the viewer could note the angle of elevation when spotting the plane but could not know its angle of elevation when the sound that was heard was made. It might be better to put the airplane directly overhead and ask for its position and angle of elevation when emitting that sound.