4050.14 – From Tangent to Sine

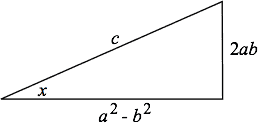
Let tan⁡(x)=2aba2−b2\tan(x) = \dfrac{2 a b}{a^2 - b^2}, where a<b<0a < b < 0 and 0<x<90∘.0 < x < 90^\circ. Draw a right triangle with angle xx and label two sides of the triangle so that tan⁡(x)=2aba2−b2.\tan(x) = \dfrac{2 a b}{a^2 - b^2}. Now find sin⁡x\sin x.

Tags:
Solution

From the figure, sin⁡(x)=2abc\sin(x) = \dfrac{2ab}{c}. But what is cc?

c2=(a2−b2)2+(2ab)2=a4−2a2b2+b4+4a2b2=a4+2a2b2+b4=(a2+b2)2\begin{aligned}c^2 = (a^2 - b^2)^2 + (2ab)^2 &= a^4 - 2a^2b^2 + b^4 + 4a^2b^2 \\&= a^4 + 2a^2b^2 + b^4 \\&= (a^2 + b^2)^2\end{aligned}

Thus c=a2+b2,c = a^2 + b^2, and sin⁡(x)=2aba2+b2.\sin(x) = \dfrac{2ab}{a^2 + b^2}.