3615.51 – Parallelepiped

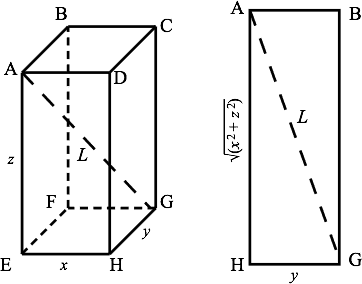
A rectangular parallelepiped has edges with integral lengths xx, yy, and zz. The sum of the lengths of all twelve edges is 72 in72 \ \inch. The sum of the areas of all six faces is 212 in2212 \ \inch^2. The volume of the solid is 144 in3144 \ \inch^3. Find the length in inches of a diagonal of this solid. And think of a reason why you might like to know this length.

Solution

We want to find the quantity L=x2+y2+z2L = \sqrt{{x^2}+{y^2}+{z^2}}.

We know that 4(x+y+z)=72⇝x+y+z=184(x + y + z) = 72 \leadsto x + y + z = 18. And that

2(xy+yz+xz)=2122(xy + yz + xz) = 212. And that xyz=144xyz = 144.

We can get all of these numbers together by considering--aha!--(x+y+z)2{(x + y + z)^2} (which equals 182=32418^2 = 324). Here we go!

(x+y+z)(x+y+z)=x2+xy+xz+yx+y2+yz+xz+zy+z2⇝x2+y2+z2+2(xy+yz+xz)=324⇝x2+y2+z2+212=324⇝x2+y2+z2=112⇝L=x2+y2+z2=112=16⋅7=47\begin{aligned}(x + y + z)(x + y + z) &=& {x^2} + xy + xz + yx + {y^2} + yz + xz + zy + {z^2} \\ &\leadsto& {x^2}+{y^2}+{z^2} + 2(xy + yz + xz) = 324 \\&\leadsto& {x^2}+{y^2}+{z^2} + 212 = 324 \\&\leadsto& {x^2}+{y^2}+{z^2} = 112 \\&\leadsto& L = \sqrt{{x^2}+{y^2}+{z^2}} = \sqrt{112} = \sqrt{16\cdot7} = 4\sqrt{7}\end{aligned}

Done.

And if you think of our parallelepiped as a box, you have just found the length of the longest cane, or sword, or stick, that will fit into it (disregarding its thickness).