3590.11 – Parallelogram and a Geometric Mean

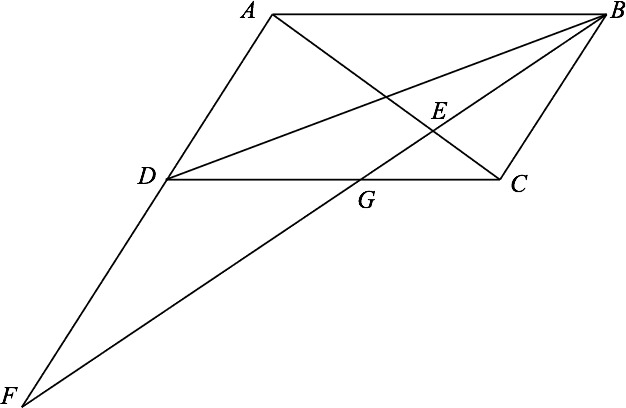
Given a parallelogram $ABCD$ with its diagonals drawn, suppose a line through $B$ intersects $AC$ at $E$ , $DC$ at $G$ , and line $AD$ extended at $F$ . Observe that triangle $AEF$ is similar to triangle $CEB$ and show that $EB$ is the geometric mean of $EG$ and $EF$ . Interested? See how this looks in a drawing.

Solution

We want $EB = \sqrt{EG \cdot EF}$ , i.e. $EB^2 = EG \cdot EF$ , i.e. $\dfrac{EF}{EB} = \dfrac {EB}{EG}$ .

We tease out some similar triangles to make this happen. There are many congruent angles to work with. Find them and use them!

First, $\triangle AEF \sim \triangle CEB$ , so $\dfrac{EF}{EB} = \dfrac{EA}{CE}$ .

Then, $\triangle CEG \sim \triangle AEB$ , so $\dfrac{EA}{CE} = \dfrac{EB}{EG}$ .

So $\dfrac{EF}{EB} = \dfrac{EB}{EG}$ , and there we are.

Hmm, what if $F = D$ ?