3440.13 – A Girl and Her Dog

Masha's dog Mitzi is tied to a corner of a walled garden with the shape of a regular hexagon 8 meters on a side. Mitzi's rope is 10 meters long and Mitzi is tied up outside the garden---usually. What is the area within which Mitzi can roam?

Answer the same question if Mitzi is tied up inside the garden.

Solution

Take a look at the figure below, particularly part (1). When Mitzi is tied outside the garden, she roams over the area ABDFJGK, which we abbreviate $I$ . When tied inside the garden, the relevant area is ABECHGK, which we call $II$ .

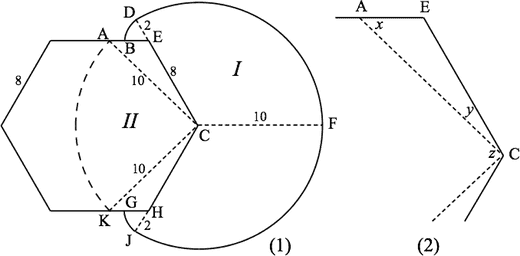
Area $I$ consists of 2/3 of a circle with radius 10 and 2/6 of a circle of radius 2. Therefore, area $I$ is

$I = \frac{2}{3} \pi 10^2 + \frac{2}{6} \pi 2^2 = 68 \pi \approx 213.6.$

This is the easy one.

Area $II$ consists of two triangles and a sector of a circle of radius 10. We need the angles $x, y$ and $z$ shown in part (2) of the figure. Remember: the vertex angle of a regular hexagon is $120°$ .

Now,

$\frac{10}{\sin 120} = \frac{8}{\sin x} \leadsto x = \arcsin \left(\frac{8 \sin 120}{10} \right) \approx 43.9°$

We next find that,

\begin{aligned} y &\approx& 180 - 120 - 43.9 = 16.1, \\ z &\approx& 120 - 2 (16.1) = 87.8. \end{aligned}

We can now find the areas relevant to $II$ :

\begin{aligned} \text{area triangle AEC } &\approx& = \frac{1}{2} 8 \cdot 10 \cdot \sin 16.1 \approx 11.1 \\ \text{area sector ACK} &\approx& \frac{87.8}{360} \pi 10^2 = 76.6. \end{aligned}

Therefore the internal roaming area for Mitzi is

$II \approx 76.6 + 2 \cdot 11.1 = 98.8.$