3420.29 – Midline Triangle

In a random triangle ABC, D is the midpoint of AB; E is the midpoint of BD; and F is the midpoint of BC.

Suppose the area of $\triangle$ ABC is $96$ . Then the area of $\triangle$ AEF is:

$16$
$24$
$32$
$36$
$48$

Solution

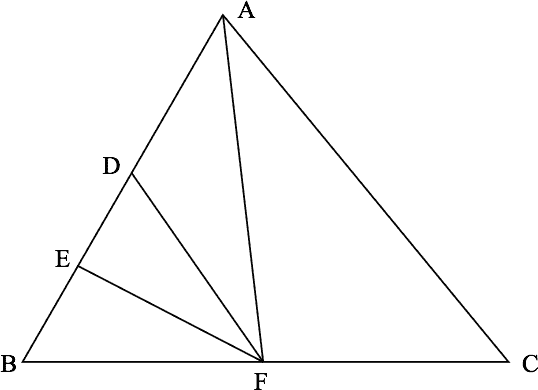
The figure described in the problem is drawn below. Note that if you halve the base of a triangle and don't change the altitude, you halve the area. Therefore,

\begin{aligned} \triangle\text{ABC} &= 96 \\ \triangle\text{ABF} &= 48 \ (\text{base being halved: BC)}\\ \triangle\text{ADF} &= 24 \ (\text{base being halved: AB)}\\ \triangle\text{BDF} &= 24 \ (\text{base being halved: AB)}\\ \triangle\text{DEF} &= 12 \ (\text{base being halved: BD)}\\ \triangle\text{AEF} &= \triangle\text{ADF} + \triangle\text{DEF} \\ &= 24 + 12 = 36. \end{aligned}