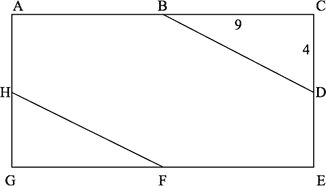
3420.26 – Area of a Hexagon

Let $B$ , $D$ , $F$ and $H$ be midpoints of the sides of a rectangle $\square ACEF$ as shown in the figure below. Find the area of hexagon $ABDEFHA$ given that $BC = 9$ and $CD = 4$ .

Solution

The area of the whole rectangle $\square ACEG$ is $8 \times 18 = 144$ . The triangles $\triangle BCD$ and $\triangle FGH$ together form a $4 \times 9$ rectangle of area 36. The area of the hexagon is $144 - 36 = 108$ .