3321.11 – A Circle and A Square

Suppose a circle and a square have the same area. What is the ratio of the area of a square inscribed inside the circle to the area of a circle inscribed inside the square?

Solution

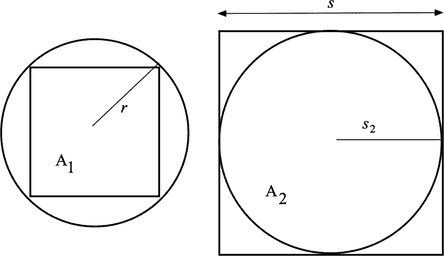
Referring to the diagram above, we are given that πrs=s2\pi r^s = s^2 so that r2=s2/πr^2 = s^2/\pi. We seek the ratio A1A2\dfrac{A_1}{A_2}. We have,

A1= half the product of the diagonals =12(2r⋅2r)=2r2A2=π(s2)2=πs24A1A2=2r2/πs24=2s2π/πs24=2s2π⋅4πs2=8π2\begin{aligned}A_1 &= \text{ half the product of the diagonals } \\&= \dfrac{1}{2} (2r \cdot 2r) = 2r^2 \\[2em]A_2 &= \pi \left( \dfrac{s}{2} \right)^2 = \dfrac{\pi s^2}{4} \\[2em]\dfrac{A_1}{A_2} &= 2r^2 / \dfrac{\pi s^2}{4} = \dfrac{2 s^2}{\pi} / \dfrac{\pi s^2}{4}\\&= \dfrac{2 s^2}{\pi} \cdot \dfrac{4}{\pi s^2} = \dfrac{8}{\pi^2}\end{aligned}