3305.51 – Making a Regular Octagon

From the plywood square shown in the figure, the corners are to be cut off to make a regular octagon. The square is $a$ inches on a side. How many inches $x$ should be cut from each corner for this purpose? Find an exact value for $x$ and also the correct value to the nearest quarter inch when $a = 12 \ \inch$ , i.e. when the plywood is a one square foot.

Solution

The diagonal sides of the octagon will be $x \sqrt{2}$ (see the figure).

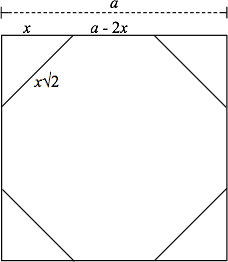
The horizontal and vertical sides will be $a - 2 x$ . Setting them equal gives:

$\begin{align*}a - 2 x &= x \sqrt{2} \\a &= 2 x + x \sqrt{2} \\&= x (2 + \sqrt{2})\end{align*}$

therefore $x = a/(2 + \sqrt{2})$ . If $a = 12$ , then

$x = \dfrac{12}{2 + \sqrt{2}} \approx 3.50$

The answer is 3.5 inches, to the nearest quarter inch.