2442.52 – Daniel Boone on Plum Creek

Daniel Boone can paddle his canoe at 15 mph in still water. However, he is paddling on Plum Creek, which, after a heavy rain, rages along at 5 mph. He paddles downriver for a while, then turns around and (with difficulty) paddles back upstream to his starting point. What is his average speed for the entire trip?

Solution

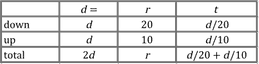
We make our trusty table (see the figure below). Note that Daniel's rates are 15 + 5 and 15 - 5.

Now we solve for $r$ :

\begin{aligned} r &=& \frac{2d}{\frac{d}{20} + \frac{d}{10}} \\ &=& \frac{2d}{\frac{3d}{20}} = 2d \cdot \frac{20}{3d} \\ &=& \frac{40}{3} = 13 \frac{1}{3} \text{ mph}. \end{aligned}

The answer is $13.33\ldots$ mi/hr—not the 15 miles per hour we might expect.