2230.81 – Cathy the Cat Burglar

One dark moonless night, in a silent city near the sea, a cubical wooden shipping crate, 1 meter by 1 meter by 1 meter, lay flat against the outside wall of a wharf-side warehouse. Midnight. From the crate crept Cathy. Noiselessly she leaned a 15\sqrt{15} meter ladder against the warehouse wall. The ladder just grazed the top of the crate before meeting the warehouse further up the wall. At what height did the ladder hit the wall?

Solution

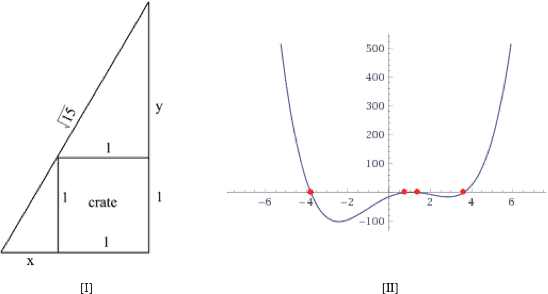
From the figure above (part [I]) we get two equations. Similar triangles gives:

y1=1x=y+1x+1\dfrac{y}{1}=\dfrac{1}{x}=\dfrac{y+1}{x+1}

and the Pythagorean Theorem gives:

(y+1)2+(x+1)2=15(y+1)^2 +(x+1)^2 =15

We want to find the quantity z=y+1z = y + 1. Using the first equation,

x+1=zz−1x + 1 = \dfrac{z}{z - 1}

therefore the second equation becomes

z2+(zz−1)2=15z^2+\left( \dfrac{z}{z-1} \right)^2 = 15

which when simplified (haw) becomes the fourth degree equation,

z4−2z3−13z2+30z−15=0z^4-2z^3-13z^2+30z-15=0

When plotted as in the figure (part [II]) we see four roots. The relevant root must be bigger than one. It appears to be approximately 3.6. It is actually 5−52\dfrac{5 - \sqrt 5}{2}.