1600.31 – Getting to Fort Wayne

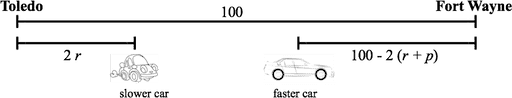
Two electric cars start out at the same time to travel from Toledo to Fort Wayne, a distance of 100 miles. They follow the same route and travel at different, although uniform, speeds, each an integral number of miles per hour. The difference in their speeds is a prime number. After driving for 2 hours, the distance of the slower car from Toledo is five times the distance of the faster car from Fort Wayne. How fast are the two cars going?

Solution

Let $r$ be the speed of the slower car and $r + p$ the speed of the faster car, where $p$ is a prime. The diagram below shows their position between the two cities after two hours. We see that

\begin{aligned} 2 r &=& 5 (100 - 2 (r + p)) \\ &=& 500 - 10 r - 10 p, \\ 10 p &=& 500 - 12 r, \\ p &=& 50 - \frac{6}{5} r. \end{aligned}

So $50 - 6 r/5$ is prime. This means that $r$ is divisible by $5$ . And this means that whatever $r/5$ is, $6$ times it is even, and thus $50 - 6r/5$ is even and thus $p$ is even. There is only one even prime, so $p = 2$ . Therefore,

\begin{aligned} 2 = 50 - \frac{6}{5} r & \leadsto & 6r = 240 \\ & \leadsto & r = 40. \end{aligned}

The two car's speeds are $40$ and $42$ .

Check: $2 \cdot 40 = 80 = 5 \cdot 16$ . Does $16$ equal $100 - 2 (42) = 100 - 84$ ? You bet!