1090.74 – How Big is This Cube?

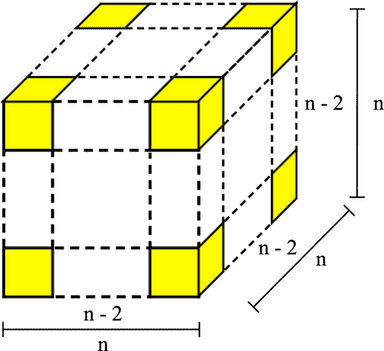
The cube in the figure is $n$ inches on a side, crafted of fine white pine and painted yellow. If it is cut into unit cubes, there will be $n^3$ of them. Each will have 0 or 1 or 2 or 3 yellow painted faces. (The drawing shows the unit cubes with 3 yellow faces.)

It happens that the number of these unit cubes with 1 yellow face is twice the number with 2 yellow faces. And the number with no yellow faces is 8 times the number with 3 yellow faces. What is $n$ ?

Solution

The unit cubes with 3 yellow faces are at the corners of the cube. There are therefore 8 of them.

The unit cubes with 2 yellow faces are located along the edges of the cube. There are 12 edges and each has $n – 2$ unit cubes with 2 yellow faces for a total of $12(n – 2)$ unit cubes with $2$ yellow faces.

The unit cubes with only one yellow face are in the interior of the $6$ faces of the cube. There are $(n – 2)^2$ such cubes in each face for a total of $6(n – 2)^2$ unit cubes with one yellow face.

Finally, the unit cubes with no yellow faces form a smaller cube inside the big cube. There are $(n – 2)^3$ of these.

The problem tells us that the number of unit cubes with 1 yellow face is twice the number of unit cubes with 2 yellow faces, and also that the number of cubes with no yellow faces is eight times the number of cubes with 3 yellow faces. That is,

$\begin{align*}6(n - 2)^2 &= 2\cdot 12(n - 2), \text{and} \\(n - 2)^3 &= 8\cdot8\end{align*}$

Now the first equation is a quadratic with the two roots: $2$ and $6$ . Only the root $6$ satisfies the second equation, so $n = 6$ is the answer.