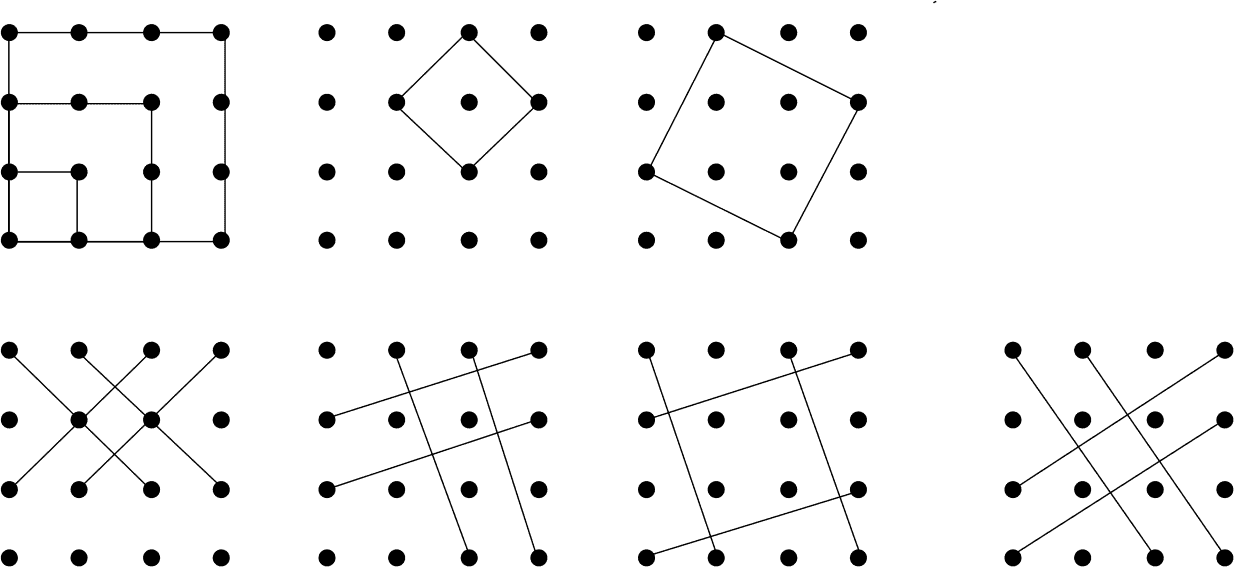
The figure shows 16 dots arranged, as it is called, in a square lattice. Say that the dots represent trees in a orchard. If you tie ropes from tree to tree you can make squares. How many different-sized squares can be created this way? You do not have to count the numbers of each kind of square, only investigate the different sized possible by tying ropes from tree to tree. Figure out good way to report what you find.

Solution
The figure below shows some of the possibilities. Some of the squares can be drawn using only one rope. These are the squares whose vertices are right at the trees. These are drawn in the first row of the figure. Others require four ropes for the four sides of the squares. Some of these are in the second row. Are there more possibilities?
There are many ways to describe, meaning to classify, the squares. Area is one. Length of side is another. Still another is by how many trees are inside the square.